In mathematics, a Taylor series is a representation of a function as an infinite sum of terms that are calculated from the values of the function's derivatives at a single point.
The concept of a Taylor series was formally introduced by the English mathematician Brook Taylor in 1715. If the Taylor series is centered at zero, then that series is also called a Maclaurin series, named after the Scottish mathematician Colin Maclaurin, who made extensive use of this special case of Taylor series in the 18th century.
It is common practice to approximate a function by using a finite number of terms of its Taylor series. Taylor's theorem gives quantitative estimates on the error in this approximation. Any finite number of initial terms of the Taylor series of a function is called a Taylor polynomial. The Taylor series of a function is the limit of that function's Taylor polynomials, provided that the limit exists. A function may not be equal to its Taylor series, even if its Taylor series converges at every point. A function that is equal to its Taylor series in an open interval (or a disc in the complex plane) is known as an analytic function.
Definition
The Taylor series of a real or complex function ƒ(x) that is infinitely differentiable in a neighborhood of a real or complex number a is the power series
which can be written in the more compact sigma notation as
where n! denotes the factorial of n and ƒ (n)(a) denotes the nth derivative of ƒ evaluated at the point a. The zeroth derivative of ƒ is defined to be ƒ itself and (x − a)0 and 0! are both defined to be 1. In the case that a = 0, the series is also called a Maclaurin series.
Examples
The Maclaurin series for any polynomial is the polynomial itself.
The Maclaurin series for (1 − x)−1 for |x| < 1 is the geometric series
so the Taylor series for x−1 at a = 1 is
By integrating the above Maclaurin series we find the Maclaurin series for log(1 − x), where log denotes the natural logarithm:
and the corresponding Taylor series for log(x) at a = 1 is
The Taylor series for the exponential function ex at a = 0 is
The above expansion holds because the derivative of ex with respect to x is also ex and e0 equals 1. This leaves the terms (x − 0)n in the numerator and n! in the denominator for each

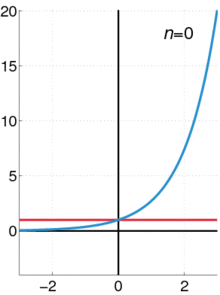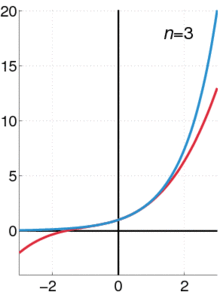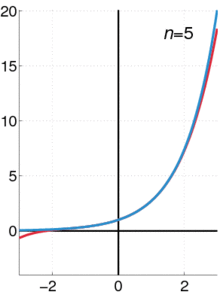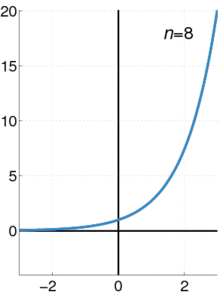







Ποτέ δεν κατάλαβα γιατι όλο ασχολούμαστε με τον Maclaurin ενώ οι σειρές είναι του Taylor..
ReplyDeleteΗ αλήθεια είναι ότι όταν δημοσίευσε το έργο του "Methodus incrementorum directa et inversa" δεν είχε υπόψιν του ότι ο Taylor τον είχε προλάβει..Το χειρότερο όλων είναι ότι ο Taylor είχε ασχοληθεί με κάτι πολύ γενικότερο..Πιστεύω ότι είναι ένα από τα πιο "πικρά ποτήρια" στην ζωή ενός επιστήμονα..
ReplyDeleteΑυτό ακριβώς!Οι Maclaurin είναι ειδική περίπτωση του Taylor...Κρίμα και άδικο...
ReplyDelete