yourMATHsolver: The Gaussian Integral

The Gaussian integral, also known as the Euler–Poisson integral is the integral of the Gaussian function e^−x² over the entire real line. It is named after the German mathematician and physicist Carl Friedrich Gauss. The integral is:

$\int_{-\infty}^\infty e^{-x^2}\,dx = \sqrt{\pi}.$

This integral has wide applications. For example, with a slight change of variables it is used to compute the normalizing constant of the normal distribution. The same integral with finite limits is closely related both to the error function and the cumulative distribution function of the normal distribution.

Although no elementary function exists for the error function, as can be proven by the Risch algorithm, the Gaussian integral can be solved analytically through the tools of calculus. That is, there is no elementary indefinite integral for $\scriptstyle\int e^{-x^2}\,dx$ , but the definite integral $\scriptstyle\int_{-\infty}^\infty e^{-x^2}\,dx$ can be evaluated.

The Gaussian integral is encountered very often in physics and numerous generalizations of the integral are encountered in quantum field theory.

Brief proof

Briefly, using the method above, one computes that on the one hand,

$\begin{align} \int_{\mathbf{R}^2} e^{-(x^2+y^2)}\,dA = \int_{-\infty}^\infty \int_{-\infty}^\infty e^{-(x^2+y^2)}\,dx\,dy\\ = \left ( \int_{-\infty}^\infty e^{-x^2}\,dx \right ) \cdot \left ( \int_{-\infty}^\infty e^{-y^2}\,dy \right )\\ = \left ( \int_{-\infty}^\infty e^{-x^2}\,dx \right )^2 \end{align}$

On the other hand,

$\begin{align} \int_{\mathbf{R}^2} e^{-(x^2+y^2)}\,dA &= \int_0^{2\pi} \int_0^{\infin} e^{-r^2}r\,dr\,d\theta\\ &= 2\pi \int_0^\infty re^{-r^2}\,dr\\ \text{Let }s = -r^2\\ &= 2\pi \int_{-\infty}^0 \frac{1}{2} e^s\,ds = \pi \int_{-\infty}^0 e^s\,ds = \pi (e^0 - e^{-\infty}) \\ & = \pi (1 - 0) = \pi, \end{align}$

where the factor of r comes from the transform to polar coordinates (r dr dθ is the standard measure on the plane, expressed in polar coordinates), and the substitution involves taking s = −r², so ds = −2r dr.

Combining these yields

$\left ( \int_{-\infty}^\infty e^{-x^2}\,dx \right )^2=\pi,$

$\int_{-\infty}^\infty e^{-x^2}\,dx=\sqrt{\pi}$ .

Careful proof

To justify the improper double integrals and equating the two expressions, we begin with an approximating function:

$I(a)=\int_{-a}^a e^{-x^2}dx.$

If the integral $\int_{-\infty}^\infty e^{-x^2}\,dx$ were absolutely convergent we would have that its Cauchy principal value, that is, the limit

$\lim_{a\to\infty} I(a)$

would coincide with $\int_{-\infty}^\infty e^{-x^2}\,dx$ . To see that this is, in fact, the case consider

$\int_{-\infty}^\infty |e^{-x^2}|\, dx < \int_{-\infty}^{-1} -x e^{-x^2}\, dx + \int_{-1}^1 e^{-x^2}\, dx+ \int_{1}^{\infty} x e^{-x^2}\, dx<\infty.$

so we can compute $\int_{-\infty}^\infty e^{-x^2}\,dx$ by just taking the limit $\lim_{a\to\infty} I(a)$ .

Taking the square of I(a) yields

$\begin{align} I(a)^2 & = \left ( \int_{-a}^a e^{-x^2}\, dx \right )\cdot \left ( \int_{-a}^a e^{-y^2}\, dy \right ) \\ & = \int_{-a}^a \left ( \int_{-a}^a e^{-y^2}\, dy \right )\,e^{-x^2}\, dx \\ & = \int_{-a}^a \int_{-a}^a e^{-(x^2+y^2)}\,dx\,dy. \end{align}$

Using Fubini's theorem, the above double integral can be seen as an area integral

$\int e^{-(x^2+y^2)}\,d(x,y),$

taken over a square with vertices {(−a, a), (a, a), (a, −a), (−a, −a)} on the xy-plane.

Since the exponential function is greater than 0 for all real numbers, it then follows that the integral taken over the square's incircle must be less than $I(a)^2$ , and similarly the integral taken over the square's circumcircle must be greater than $I(a)^2$ . The integrals over the two disks can easily be computed by switching from cartesian coordinates to polar coordinates:

$\begin{align} x & = r \cos \theta \\ y & = r \sin\theta \\ d(x,y) & = r\, d(r,\theta). \end{align}$

$\int_0^{2\pi}\int_0^a re^{-r^2}\,dr\,d\theta < I^2(a) < \int_0^{2\pi}\int_0^{a\sqrt{2}} re^{-r^2}\,dr\,d\theta.$

(See to polar coordinates from Cartesian coordinates for help with polar transformation.)

Integrating,

$\pi (1-e^{-a^2}) < I^2(a) < \pi (1 - e^{-2a^2}).$

By the squeeze theorem, this gives the Gaussian integral

$\int_{-\infty}^\infty e^{-x^2}\, dx = \sqrt{\pi}.$

By Cartesian coordinates

A different technique, which goes back to Laplace (1812), is the following.

Let

$\begin{align} y & = xs \\ dy & = x\,ds. \end{align}$

Since the limits on $s$ as $y$ goes to $\pm\infty$ depend on the sign of $x$ , it simplifies the calculation to use the fact that $e^{-x^2}$ is an even function, and, therefore, the integral over all real numbers is just twice the integral from zero to infinity. That is, $\int_{-\infty}^{\infty} e^{-x^2}\,dx = 2\int_{0}^{\infty} e^{-x^2}\,dx$ . Thus, over the range of integration, $x\ge 0$ , and the variables $y$ and $s$ have the same limits. This yields:

$I^2 = 4 \int_0^\infty \int_0^\infty e^{-(x^2 + y^2)} dy\,dx .$

Then

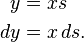
$\begin{align} \frac{I^2}{4} & = \int_0^\infty \left( \int_0^\infty e^{-(x^2 + y^2)} \, dy \right) \, dx = \int_0^\infty \left( \int_0^\infty e^{-x^2(1+s^2)} x\,ds \right) dx \\[5pt] & = \int_0^\infty \left( \int_0^\infty e^{-x^2(1 + s^2)} x \, dx \right) \, ds \\[5pt] & = \int_0^\infty \left[ \frac{1}{-2(1+s^2)} e^{-x^2(1+s^2)} \right]_0^\infty \, ds = \frac{1}{2} \int_0^\infty \frac{ds}{1+s^2} \\[5pt] & = \frac{1}{2} \left. \arctan s \frac{}{} \right|_0^\infty = \frac{\pi}{4}. \end{align}$